-
My ToshibaSemicon
- General Top View
-
SEMICONDUCTOR View
-
ApplicationsAutomotive
Body Electronics
xEV
In-Vehicle Infotainment
Advanced Driver-Assistance Systems (ADAS)
Chassis
IndustrialInfrastructure
BEMS/HEMS
Factory Automation
Commercial Equipment
Consumer/PersonalIoT Equipment
Healthcare
Wearable Device
Mobile
Computer Peripherals
-
ProductsAutomotive Devices
Discrete Semiconductor
Diodes
Transistors
Logic ICs
Analog Devices
Digital Devices
Wireless Devices
※
: Products list (parametric search)
Power SemiconductorsSiC Power Devices
※
: Products list (parametric search)
Isolators/Solid State RelaysPhotocouplers
Digital Isolators
Solid State Relays
Fiber Optic Transmitting Modules
※
: Products list (parametric search)
MOSFETsIGBTs/IEGTsBipolar Transistors※
: Products list (parametric search)
Diodes※
: Products list (parametric search)
MicrocontrollersMotor Driver ICsIntelligent Power ICs※
: Products list (parametric search)
Power Management ICsLinear ICs※
: Products list (parametric search)
General Purpose Logic ICsLinear Image SensorsOther Product ICsOther Product ICs
※
: Products list (parametric search)
-
Design & Development
Design & Development
Innovation Centre
At the Toshiba Innovation Centre we constantly strive to inspire you with our technologies and solutions. Discover how to place us at the heart of your innovations.
-
Knowledge
Knowledge
Highlighted Topics
Further Materials
Other
- Where To Buy View
-
My ToshibaSemicon
-
- STORAGE View
- COMPANY View
- Part Number Search
- Cross Reference Search
- Keyword Search
- Parametric Search
- Stock Check & Purchase
This webpage doesn't work with Internet Explorer. Please use the latest version of Google Chrome, Microsoft Edge, Mozilla Firefox or Safari.
require 3 characters or more.
The information presented in this cross reference is based on TOSHIBA's selection criteria and should be treated as a suggestion only. Please carefully review the latest versions of all relevant information on the TOSHIBA products, including without limitation data sheets and validate all operating parameters of the TOSHIBA products to ensure that the suggested TOSHIBA products are truly compatible with your design and application.Please note that this cross reference is based on TOSHIBA's estimate of compatibility with other manufacturers' products, based on other manufacturers' published data, at the time the data was collected.TOSHIBA is not responsible for any incorrect or incomplete information. Information is subject to change at any time without notice.
require 3 characters or more.
2.2. Open-loop and closed-loop gains (Increasing the bandwidth of an amplifier)
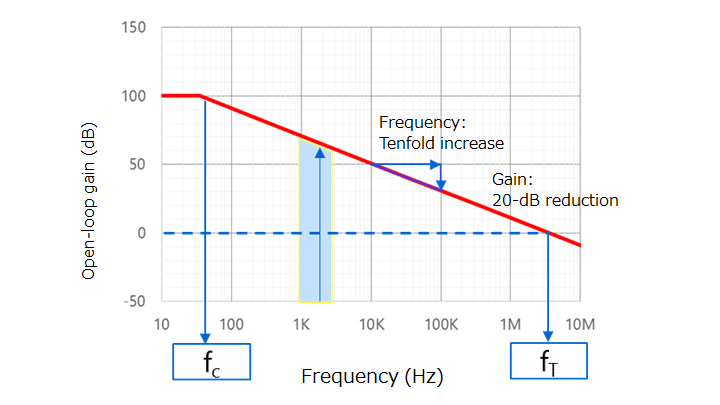
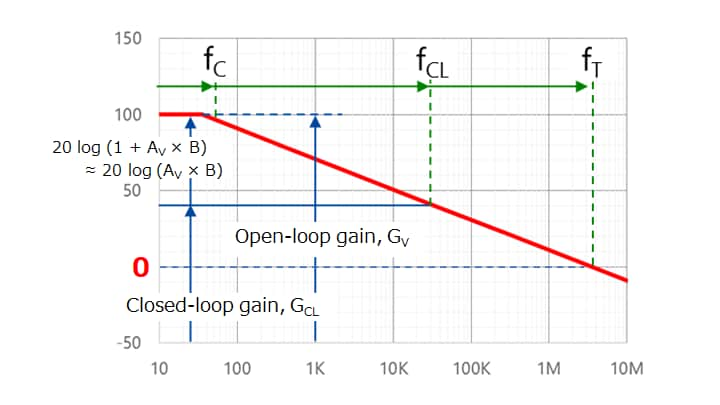
The open-loop gain (GV) of an op-amp has the same frequency characteristics as a first-order RC lowpass filter as shown in Figure 2-3. At frequencies higher than the corner frequency (fC) at which the open-loop gain is 3 dB lower than the DC gain, the open-loop gain decreases at a rate of 6 dB per octave (20 dB per decade). In this frequency range, the decibel open-loop gain of the op-amp (GV) decreases by 6 dB (i.e., the linear open-loop gain (AV) halves) when the frequency doubles. Hence:
fc × AV = constant
The frequency at which the gain is equal to 1 (0 dB) is called the unity gain cross frequency (fT). Therefore, the above equation can be restated as follows. This is called the gain-bandwidth product (designated as GBWP, GBW, GBP, or GB).
fc × AV = fT
Note that this equation is true in the frequency range in which the open-loop gain decreases at a rate of 6 dB per octave.
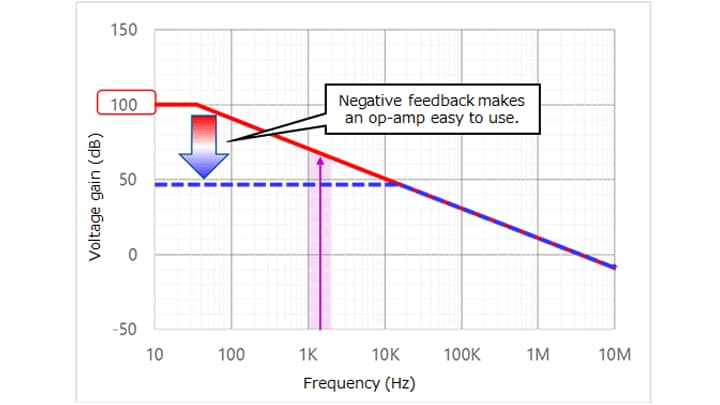
Now, let’s consider what occurs when an input signal with a frequency of 2±1 kHz is applied to an op-amp that has frequency characteristics as shown in Figure 2-3. In the case of an op-amp under this condition, the gain at 3 kHz is roughly 10 dB lower than the gain at 1 kHz. Normally, the op-amp cannot be used under this condition. Negative feedback solves this issue.
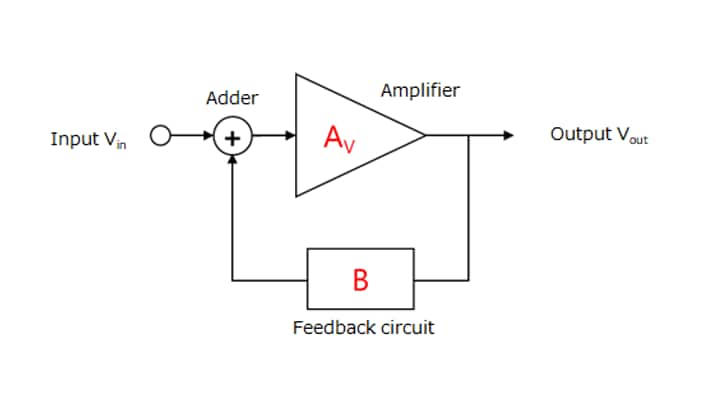
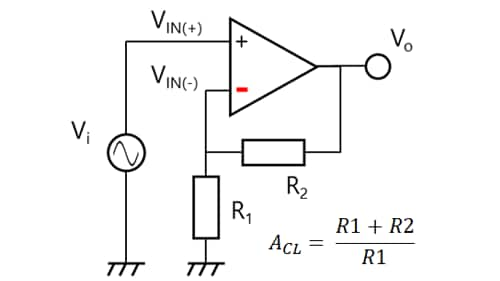
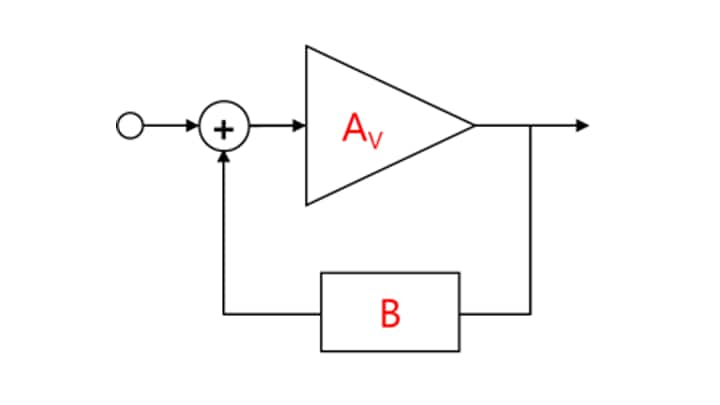
The input (Vin) and the output (Vout) have the following relationship. This relationship is called a closed-loop gain (represented as GCL in dB scale and ACL in linear scale). The 20 log rule is used to convert a linear voltage gain into a decibel voltage gain: G = 20 × log A.
Vout / Vin = ACL = AV / (1 + AV × B)
= 1 / {B (1 + 1 / AV × B)}
where AV is the open-loop gain of an amplifier and B is the feedback factor. (AV × B) is called the loop gain. The denominator, (1 + AV × B), is called the amount of feedback. In the case of negative feedback, AV × B < 0. An op-amp has a very high AV. Hence, | AV x B | >> 1. Therefore, the amount of feedback is calculated as (1 + AV × B) ≈ AV × B (loop gain). Hence, the above equation can be simplified as follows:
Vout / Vin = ACL = 1/B
Figure 2-5 shows this relationship. The op-amp has a bandwidth of fC. With negative feedback, its closed-loop bandwidth expands to fCL. fCL is calculated as follows from the gain-bandwidth product equation:
fCL = fT / ACL
When the closed-loop gain (GCL) or the bandwidth (fCL) is insufficient, it is necessary to select an op-amp with high fT.
Related information
Related information
- Products
- Application Notes
- FAQs
- Parametric Search
- Stock Check & Purchase