This page partially uses JavaScript. This page may not operate normally when these functions are not supported by your browser or the setting is disabled.
Please look for the information you need from the following pages:

Asia-Pacific - English

Asia-Pacific - 简体中文

Asia-Pacific - 繁體中文

Asia-Pacific - 한국어

Asia-Pacific - 日本語

Americas - English

Europe (EMEA) - English

2.3. Oscillation

Figure 2-6 Growth of an unwanted signal through a negative feedback circuit

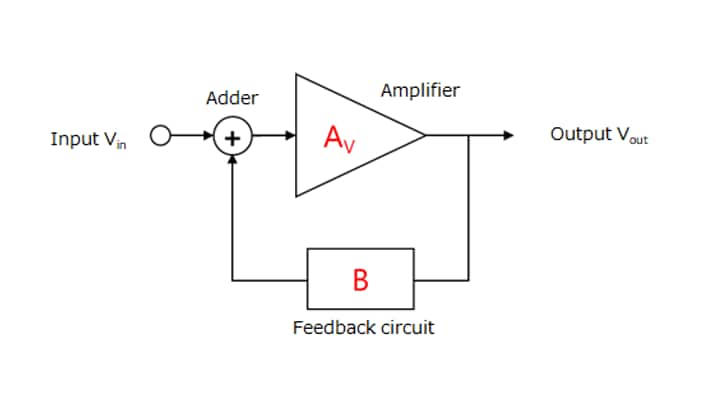
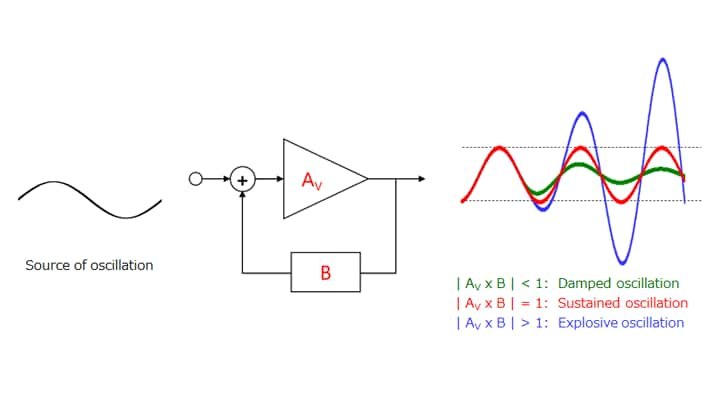
An op-amp is normally used with a feedback circuit as shown in Figure 2-6. There are two types of feedback, positive and negative, as described in Section 2.1. When an op-amp is used as an amplifier, it is configured for negative feedback. Care is required as to the oscillation of a feedback circuit.
A signal or noise that acts as a source of oscillation can develop into oscillation under certain conditions. Here is a brief explanation about oscillation.
A source of oscillation applied to the input passes through the amplifier and the feedback circuit. Then, the adder adds it to the V_in input. Therefore, the output of the adder becomes larger than the initial state. As this process is repeated, the source of oscillation grows, causing oscillation. This is the characteristic of positive feedback.
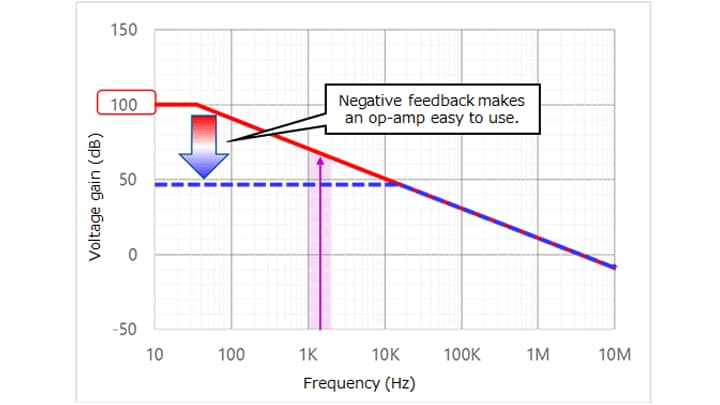
You might think that oscillation is irrelevant as you use negative feedback . Even if it is negative feedback for the signal to be amplified, it might turn into positive feedback in the higher frequency band.

Figure 2-7 Oscillation generated by a feedback circuit

Let the open-loop gain of the op-amp be A_V and the feedback factor be B. Then, the transfer function of a feedback circuit is expressed as follows. Both A_V and B are complex numbers.

V_out = A_V / (1 + A_V × B) × V_in

In the case of a negative feedback circuit, A_V × B ＝ +|A_V × B|. Therefore, V_out provides a stable output as described in Section 2.1. However, the phase of the output lags that of the input at high frequency since all circuits have a delay.
The feedback circuit turns into a positive feedback loop when this phase lag reaches 180 degrees.
The waveform of the output (V_out) differs as shown below, depending on the magnitude of the loop gain (|A_V x B|) of positive feedback (i.e., when the signal from the feedback circuit has the same phase as the input signal). When a source of oscillation is applied to the input, a damped, sustained, or explosive oscillation occurs, depending on the magnitude of |A_V × B| at the frequency of the oscillation source. Generally, a sustained oscillation is called an oscillation. An explosive oscillation eventually subsides to a sustained oscillation since the open-loop gain (A_V) is restricted by the dynamic range of the amplifier.

Figure 2-8 First-order delay due to the main pole

A sustained oscillation occurs when the loop gain (A_V × B) satisfies the following condition (i.e., the denominator of the transfer function becomes zero). This condition is called the Barkhausen condition for oscillation (or simply oscillation condition).

Amplitude condition: R_e (A_V × B) = -1
Phase condition: I_m (A_V × B) = 0

Note that an explosive oscillation eventually subsides to a sustained oscillation as described above. Therefore, the amplitude condition that causes abnormal oscillation is as follows:

Amplitude condition: R_e (A_V × B) < -1

Because of an internal parasitic capacitance, an op-amp has a first-order delay element (as is the case with a first-order lowpass filter) as shown in Figure 2-8.
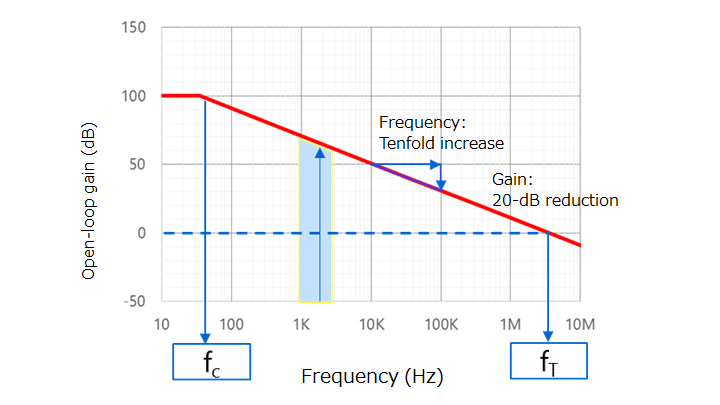
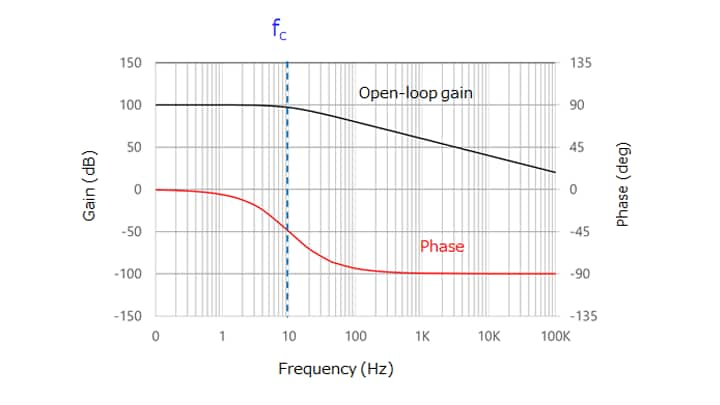
In the case of typical op-amps, the cut-off frequency of open-loop gain response is between 10 Hz and 100 Hz. The phase of the output lags 45 degrees behind in this frequency range. The phase lag is 90 degrees in the frequency range in which the open-loop gain decreases at a rate of 6 dB per octave.
If the gain-vs-frequency curve has such characteristics with only a main pole, a margin of 90 degrees remains until oscillation occurs. Therefore, oscillation is unlikely to occur.

Figure 2-9 Gain and phase curves including a second pole

In reality, an op-amp has multiple poles. The cut-off frequency (f_c) shown in Figure 2-9 is called the main pole. The frequency pole at f_c2 close to the unity gain cross frequency (f_T) is called a second pole. Although there are more poles at higher frequencies, they do not cause any practical problem.

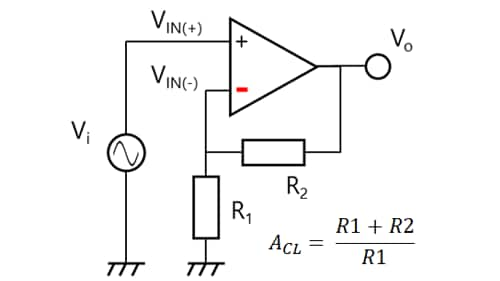
As shown in Figure 2-9, the slope of the open-loop gain curve changes from 6 dB per octave to 12 dB per octave at f_c2. The phase lag also increases further by another 45 degrees. This phase lag does not cause any problem when f_c2 is higher than the unity gain cross frequency (f_T). However, even when f_c2 is lower than f_T, care should be taken when using op-amps as unity gain amplifiers such as voltage followers. (If the datasheet for an op-amp states that it can be used with unity gain, it has a second pole at a frequency higher than f_T.)
In order to avoid abnormal oscillation, an op-amp should be used in the frequency range (f_c to f_c2) in which the open-loop gain decreases at a rate of 6 dB per octave. Note, however, that, at a frequency close to f_c2, an op-amp is affected by a second pole, causing a power loss and a phase delay. To avoid its effects completely, the closed-loop bandwidth (f_CL) should be less than one-fifth of f_c2.

The above is a discussion on the oscillation of an op-amp itself.
It is also necessary to ensure that external circuitry is also free from oscillation (e.g., phase delay).
For example, this consideration applies to an application in which a capacitive load is driven by an op-amp. Oscillation occurs if the cut-off frequency due to the capacitive load is within the range in which the loop gain is greater than 1. In order to prevent oscillation, it is necessary, for example, to add a resistor in series with a capacitor. Even when an op-amp is not connected with a load, care should be taken as to wire or other capacitance. Minimize the length of the wire from the op-amp output to the subsequent device and that of the feedback loop.